Last updated on
Nov 13, 2024
Complete Ordered Field For Free
Drop document here to upload
Up to 100 MB for PDF and up to 25 MB for DOC, DOCX, RTF, PPT, PPTX, JPEG, PNG, JFIF, XLS, XLSX or TXT
Note: Integration described on this webpage may temporarily not be available.
0
Forms filled
0
Forms signed
0
Forms sent
Try these PDF tools
Edit PDF
Quickly edit and annotate PDFs online.
Start now
Sign
eSign documents from anywhere.
Start now
Request signatures
Send a document for eSignature.
Start now
Share
Instantly send PDFs for review and editing.
Start now
Merge
Combine multiple PDFs into one.
Start now
Rearrange
Rearrange pages in a PDF document.
Start now
Compress
Compress PDFs to reduce their size.
Start now
Convert
Convert PDFs into Word, Excel, JPG, or PPT files and vice versa.
Start now
Discover the simplicity of processing PDFs online
Upload your document in seconds
Fill out, edit, or eSign your PDF hassle-free
Download, export, or share your edited file instantly
Top-rated PDF software recognized for its ease of use, powerful features, and impeccable support
Every PDF tool you need to get documents done paper-free
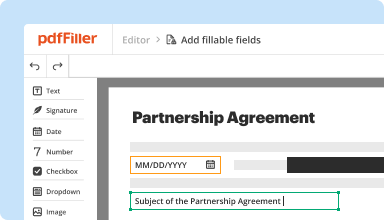
Create & edit PDFs
Generate new PDFs from scratch or transform existing documents into reusable templates. Type anywhere on a PDF, rewrite original PDF content, insert images or graphics, redact sensitive details, and highlight important information using an intuitive online editor.
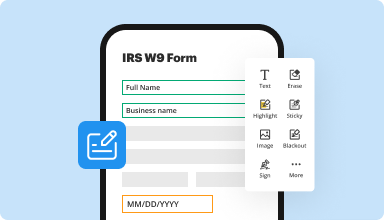
Fill out & sign PDF forms
Say goodbye to error-prone manual hassles. Complete any PDF document electronically – even while on the go. Pre-fill multiple PDFs simultaneously or extract responses from completed forms with ease.
Organize & convert PDFs
Add, remove, or rearrange pages inside your PDFs in seconds. Create new documents by merging or splitting PDFs. Instantly convert edited files to various formats when you download or export them.
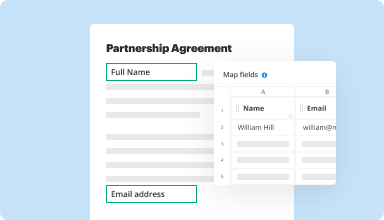
Collect data and approvals
Transform static documents into interactive fillable forms by dragging and dropping various types of fillable fields on your PDFs. Publish these forms on websites or share them via a direct link to capture data, collect signatures, and request payments.
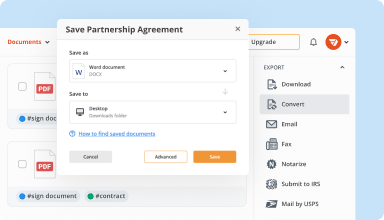
Export documents with ease
Share, email, print, fax, or download edited documents in just a few clicks. Quickly export and import documents from popular cloud storage services like Google Drive, Box, and Dropbox.
Store documents safely
Store an unlimited number of documents and templates securely in the cloud and access them from any location or device. Add an extra level of protection to documents by locking them with a password, placing them in encrypted folders, or requesting user authentication.
Customer trust by the numbers
64M+
users worldwide
4.6/5
average user rating
4M
PDFs edited per month
9 min
average to create and edit a PDF
Join 64+ million people using paperless workflows to drive productivity and cut costs
Why choose our PDF solution?
Cloud-native PDF editor
Access powerful PDF tools, as well as your documents and templates, from anywhere. No installation needed.
Top-rated for ease of use
Create, edit, and fill out PDF documents faster with an intuitive UI that only takes minutes to master.
Industry-leading customer service
Enjoy peace of mind with an award-winning customer support team always within reach.
What our customers say about pdfFiller
See for yourself by reading reviews on the most popular resources:
trying to find the right form that was not already filled out.
Also you need to add the ability to erase to the lowest membership level. You can do a couple of things such as restrict the number of new forms per month, offer a 1-5 form only fee, or only a 1 month membership fee. In the past 3 years I've used this service only twice now as I am not a business.
2015-01-26
This has been very helpful. My only problem is a tried to sign up for a monthly membership and I ended up getting charged and receiving a year membership. That part is a bit confusing.
2016-11-01
The product is excellent, but very expensive for an occasional user. I paid a lot of money just to download two documents. Surely it would not be difficult to categorise number of forms required, and a calculated price accordingly? I will probably not require another form for 12 months or more. Unfortunately, it leaves a bad taste in the mouth.
2018-07-25
What do you like best?
The ease of downloading our own files to manipulate the data, and/or recreate a document.
What do you dislike?
I think a better training tool/video would be beneficial for new and old users.
Recommendations to others considering the product:
Great product and easy to use.
What problems are you solving with the product? What benefits have you realized?
We are able to recreate documents and manipulate the information needed in order to prepare real estate documents at a much faster pace.
The ease of downloading our own files to manipulate the data, and/or recreate a document.
What do you dislike?
I think a better training tool/video would be beneficial for new and old users.
Recommendations to others considering the product:
Great product and easy to use.
What problems are you solving with the product? What benefits have you realized?
We are able to recreate documents and manipulate the information needed in order to prepare real estate documents at a much faster pace.
2018-12-31
What do you like best?
The ability to sign, fill out and send documents easily and quickly.
What do you dislike?
Slow start up.and slow "save as" time. That is all.
Recommendations to others considering the product:
none at this time
What problems are you solving with the product? What benefits have you realized?
Signing contracts.
The ability to sign, fill out and send documents easily and quickly.
What do you dislike?
Slow start up.and slow "save as" time. That is all.
Recommendations to others considering the product:
none at this time
What problems are you solving with the product? What benefits have you realized?
Signing contracts.
2018-12-31
Time saver!
I have used PDFfiller for several months now and love it. It is a great tool which makes it extremely easy for me to quickly and efficiently fill out PDFs and return them to the sender. Wether it be filling out forms or signing a contract, it has saved me many many hours in the long run - and is a pleasure to use!
There aren't really any cons to this plugin - it does exactly what it says it will do. However the user interface could be improved
2018-11-06
Not sure how I "subscribed", yet PDFfiller honoured their policy of full cancellation and FULL REFUND.
I'm IMPRESSED !!!
The went further and added an offer of a further FREE TRIAL.
If their products are as good as their service, I'll definitely intentionally subscribe at some stage in the future ... after South Africa's political siege they call "Lockdown".
Well done PDFfiller.
2020-06-30
Would recommend 100%
This app is really great and offers I good service. I had an issue and contacted the customer service, they resolved it within an hour and were super amiable
2020-05-13
As an accountant
As an accountant, even one who does not prepare taxes, this has turned out to be a life-saver for me with all the POA's and other forms that need to be filled out and signed. Thanks pdfFiller!
2025-03-14
How-to Guide
How to edit a PDF document using the pdfFiller editor:
01
Download your form to the uploading pane on the top of the page
02
Find the Complete Ordered Field feature in the editor's menu
03
Make all the needed edits to the file
04
Push “Done" button to the top right corner
05
Rename the template if needed
06
Print, download or share the file to your computer
Complete Ordered Field Feature
The Complete Ordered Field feature enhances input management by providing a structured way to organize data. It offers precision and clarity, allowing you to maintain control over your data sets efficiently.
Key Features
Ensures consistent data entry
Supports customization for various applications
Facilitates easy integration with existing systems
Improves data reliability and accuracy
Enables quick retrieval of information
Use Cases and Benefits
Data entry in customer relationship management systems
Organizing product information in e-commerce platforms
Managing inventory in supply chain operations
Maintaining accurate records in healthcare settings
Streamlining project management tasks
This feature addresses common data organization challenges. By using Complete Ordered Field, you can prevent data entry errors, save time on data management, and enhance overall productivity. Experience smooth data handling that meets your needs.
For pdfFiller’s FAQs
Below is a list of the most common customer questions. If you can’t find an answer to your question, please don’t hesitate to reach out to us.
What if I have more questions?
Contact Support
Are the rationals an ordered field?
By Rational Numbers form Field, (Q, +,×) is a field. By Total Ordering on Quotient Field is Unique, it follows that (Q, +,Ã) has a unique total ordering on it that is compatible with its ring structure. Thus, (Q,+,Ã,) is a totally ordered field.
Can the complex numbers be ordered?
TL;DR: The complex numbers are not an ordered field; there is no ordering of the complex numbers that is compatible with addition and multiplication. If a structure is a field and has an ordering, two additional axioms need to hold for it to be an ordered field.
What is the field Q?
A field consists of a set of elements together with two operations, namely addition, and multiplication, and some distributivity assumptions. A prominent example of a field is the field of rational numbers, commonly denoted Q, together with its usual operations of addition and multiplication.
What is a field in real analysis?
In mathematics, a field is a set on which addition, subtraction, multiplication, and division are defined and behave as the corresponding operations on rational and real numbers do. The best known fields are the field of rational numbers, the field of real numbers and the field of complex numbers.
How do you prove something is an ordered field?
A field (F, +,) together with a (strict) total order < on F is an ordered field if the order satisfies the following properties for all a, b and c in F: if a < b then a + c < b + c, and. If 0 < a and 0 < b then 0 < ab.
What is an example of a field?
The set of real numbers and the set of complex numbers each with their corresponding + and * operations are examples of fields. However, some non-examples of a field include the set of integers, polynomial rings, and matrix rings.
What is a field?
In physics, a field is a physical quantity, represented by a number or tensor, that has a value for each point in space-time. In the modern framework of the quantum theory of fields, even without referring to a test particle, a field occupies space, contains energy, and its presence precludes a classical “true vacuum".
What makes a field?
A field is a set F, containing at least two elements, on which two operations. + and · (called addition and multiplication, respectively) are defined so that for each pair. Of elements x, y in F there are unique elements x + y and x · y (often writteXYxy) in F for.
Are the integers a field?
Field. A familiar example of a field is the set of rational numbers and the operations addition and multiplication. An example of a set of numbers that is not a field is the set of integers. It is an “integral domain." It is not a field because it lacks multiplicative inverses.
Are real numbers a field?
In mathematics, a field is a set on which addition, subtraction, multiplication, and division are defined and behave as the corresponding operations on rational and real numbers do. The best known fields are the field of rational numbers, the field of real numbers and the field of complex numbers.
Is R an ordered field?
Any set which satisfies all eight axioms is called a complete ordered field. We assume the existence of a complete ordered field, called the real numbers. The real numbers are denoted by R.
Can complex numbers be ordered?
In fact, there is no linear ordering on the complex numbers that is compatible with addition and multiplication the complex numbers cannot have the structure of an ordered field. This is because any square in an ordered field is at least 0, but i2 = 1.
How do you determine if a set is a field?
A set can't be a field unless it's equipped with operations of addition and multiplication, so don't ask unless it has those specified. If a set has specified operations of addition and multiplication, then you can ask if with those operations it is a field. Just check to see if it satisfies the axioms of a field.
Why are complex numbers not ordered?
TL;DR: The complex numbers are not an ordered field; there is no ordering of the complex numbers that is compatible with addition and multiplication. If a structure is a field and has an ordering, two additional axioms need to hold for it to be an ordered field.
Are the complex numbers an ordered field?
Every ordered field is a formally real field, i.e., 0 cannot be written as a sum of nonzero squares. The complex numbers also cannot be turned into an ordered field, as 1 is a square (of the imaginary number i) and would thus be positive.
#1 usability according to G2
Try the PDF solution that respects your time.